
Relativity Myths & Ideas
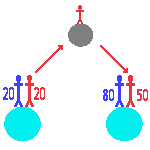
Scenarios - The Twin Paradox
Under Special Relativity, motion at a constant velocity is relative and relative motion gives rise to the dilation of time (as described by the Lorentz transformation). If the time dilation is taken to be symmetrical, it leads to the impression that there is a paradox, as is illustrated by the "Twin Paradox" thought experiment...
There are two twins, one of whom stays on the Earth. The other twin travels in a spaceship at near-light velocity to a distant star. The spaceship then turns round and travels back to the Earth. From the point of view of the twin on Earth, less time would pass for the traveller than for himself. So when he returned, the traveller would be younger than the twin on Earth. Indeed if the traveller traveled at 0.866 c (86.6% of the speed of light) he would experience half the time elapse of his twin. So if they were 20 years old at the start, and the traveller was 50 when he returned, the twin who stayed on the Earth would be 80 years old.
However, If the time dilation is symmetrical, then, from the point of view of the traveller, he is stationary in his rest frame and the Earth, and his twin, recede very fast from him, then return. So, for the traveller, the twin on Earth should experience less time passing. I.e. the traveller should be 80 and the twin on Earth 50 years old!
Paul Langevin's Explanation of the Paradox
It is clear that the assumption that the time dilation is symmetrical leads to an impossible situation, hence the paradox. It is this assumption that Paul Langevin addressed in 1911. He suggested that, as the traveller underwent acceleration at the beginning, turnaround and end of the journey, his rest frame was not inertial. Whereas, the twin on Earth did not undergo acceleration, therefore his rest frame was inertial. Thus the situation is not symmetrical. In effect, this suggests that the traveller was actually moving with respect to the Earth.
Note: The asymmetry is not intended to imply the existence of an absolute state of rest against which the motion occurs. It merely describes an asymmetry in the motion of the traveller with respect to the Earth.
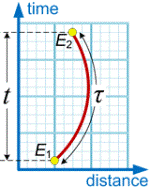
A Modern Explanation of the Twin Paradox
This explanation is based on a Wikipedia article on Proper Time:
In relativity, proper time is the elapsed time between two events as measured by a clock that passes through both events. The proper time depends not only on the events but also on the motion of the clock between the events. An accelerated clock will measure a smaller elapsed time between two events than that measured by a non-accelerated (inertial) clock between the same two events. The twin paradox is an example of this effect.
The dark blue vertical line represents an inertial observer measuring a coordinate time interval t between events E1 and E2. The red curve represents a clock measuring its proper time T between the same two events.
In terms of four-dimensional spacetime, proper time is analogous to arc length in three-dimensional (Euclidean) space. By convention, proper time is usually represented by the Greek letter T (tau) to distinguish it from coordinate time represented by t.
So far, so good, however, the article goes on to say...
By contrast, coordinate time is the time between two events as measured by a distant observer using that observer's own method of assigning a time to an event. In the special case of an inertial observer in special relativity, the time is measured using the observer's clock and the observer's definition of simultaneity.
Whilst this description is sufficient for the purpose of explaining the twin paradox, it may give the impression that proper time and coordinate time are separate measures of time. This is not so. As we note here, coordinate time is the time that a clock measures between two events irrespective of whether it is co-located with them. So it includes the circumstances in which it is co-located. Hence, proper time is a sub-set of coordinate time.
Further details of the twin paradox, including a worked example, may be found here.
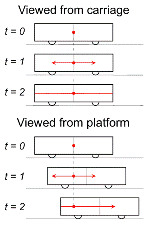
Einstein's Train
This version of the Einstein's Train scenario is based on an account in Wikipedia. It seeks to explain how differences in simultaneity arise:
A strobe light mounted in the middle of a railway carriage sends out a single pulse towards the front and the back of the carriage. To an observer on the train the ends of the carriage are an equal distance from the source of the light, and the light approaches both at the same speed. So the light reaches the front and the back of the carriage simultaneously.
To an observer on the platform, the light is moving towards the back and front of the carriage at equal speeds, but because the carriage is moving, the back of the carriage is approaching the light, whilst the front is receding. So the light travelling to the back of the carriage has less distance to travel than the light travelling to the front. So the light reaches the back of the carriage first.
Hence events which are simultaneous to the observer on the train are not simultaneous to the observer at the station. That's a summary of the explanation given in Wikipedia, and it seems to suggest that the difference in simultaneity is a real, measurable, occurrence. However, there are reasons to question this view:
- The difference in simultaneity has never actually been empirically shown to exist. It only arises between events that are not, and cannot, be causally related, so it may be impossible to prove whether it exsts or not anyway.
- We question here whether time dilation and differences in simultaneity are real phenomena.
- Dr John Field, who is a former researcher at CERN, has reworked Einstein's derivation of the Lorentz transformation. His work, which is summarised here, suggests that differences in the elapse of time between events arise without a difference in simultaneity.
We pursue these matters in the Two Towers example. However, we have another scenario to consider first...

The Andromeda Paradox
The Andromeda Paradox is similar to the Einstein's Train s but extends the concept of differences in simultaneity into planes of simultaneity which extend throughout spacetime. These are thought to be affected by the velocity and direction of motion of the observer, and the distance between the observer and an object.
All these factors combine, so if the observer A has a small velocity towards a very distant object (such as Andromeda), this can move the plane of simultaneity such that events in the future of Andromeda (e.g. the launch of an invasion fleet of spaceships towards Earth) become simultaneous with events in the present local to the observer.
Similarly, if observer B has a small velocity away from the object (Andromeda), this moves the plane of simultaneity into the past (e.g. before the decision to send the fleet was made). Thus in B's present, the spaceships have not been launched. A fuller explanation of the paradox is given here.
This idea may arise from a fundamental misunderstanding of the nature of spacetime. In particular, that a zero spacetime interval between two events means that they are simultaneous, and a non-zero interval means that they are not. We deal with that in more detail under Zero Intervals, but here it is sufficient to note that simultaneous events will only have a zero spacetime interval if they are co-located. If they are separated spatially, they will have a non-zero "spacelike" spacetime interval. Likewise, events that are not simultaneous can have a zero spacetime interval in appropriate circumstances. It follows that conclusions about the simultaneity of events based solely upon events having a zero spacetime interval are spurious.
The Two Towers
The Two Towers
The Two Towers is a thought experiment that illustrates the problems in interpreting the differences in simultaneity that arise when viewing an event from different rest frames.
The west and east Elysian Towers are derelict 1960's tower blocks in the north of England. They are due to be demolished, and charges are rigged so that the towers will fall simultaneously towards each other, making a neat pile of rubble, and no one will get hurt. And that's what happens, well at least so far as local spectators are concerned. The charges go off simultaneously, the towers fall towards each other, they end up in a neat pile in the middle, and no one gets hurt. Job done.
But according to special relativity, the simultaneity of events is dependent on the frame of reference in which it is observed. So what would be observed on a spaceship from Andromeda that is travelling at near-light velocity in the direction from the west tower eastwards? Would the pilot see the west tower fall before the east tower, spilling rubble beside the east tower and killing some spectators?
Then there is another spaceship from Andromeda travelling past the Earth in the opposite direction, from east to west. Would this pilot see rubble spill out beside the west tower, killing different spectators? Are there three different versions of events? No. That is, of course, nonsense, and is not what Special Relativity predicts. No matter how many spaceships fly in different directions and have different views of it, what happens happens.
So how real are the differences in simultaneity? It is apparent that, in reality, the differences in simultaneity have no effect on the outcome. Furthermore they cannot have any effect, because the relationship between the spaceships flying past and the towers being demolished is space-like. Space-like relationships cannot have causal relationships. The one event simply cannot affect the other.
Also, although it is true to say that there is a lot of empirical evidence that supports the theory of relativity, differences in simultaneity have never been empirically shown to exist. So although the idea of a difference in simultaneity is integral to the theory, there is no reason to deduce that they actually exist. They could be no more than a mathematical anomaly produced by the way that Einstein derived the theory.
